报告人:李亚光 多伦多大学 博士后
报告时间:12月5号上午 9:00-9:30
报告地点:腾讯会议 ID 504 790 537
报告题目:A model‐based multithreshold method for subgroup identification
摘要:Thresholding variable plays a crucial role in subgroup identification for personalized medicine. Most existing partitioning methods split the sample based on one predictor variable. In this paper, we consider setting the splitting rule from a combination of multivariate predictors, such as the latent factors, principle components, and weighted sum of predictors. Such a subgrouping method may lead to more meaningful partitioning of the population than using a single variable. In addition, our method is based on a change point regression model and thus yields straight forward model‐based prediction results. After choosing a particular thresholding variable form, we apply a two‐stage multiple change point detection method to determine the subgroups and estimate the regression parameters. We show that our approach can produce two or more subgroups from the multiple change points and identify the true grouping with high probability. In addition, our estimation results enjoy oracle properties. We design a simulation study to compare performances of our proposed and existing methods and apply them to analyze data sets from a Scleroderma trial and a breast cancer study.
个人简介: 李亚光,统计学博士。2018年11月在中国科学技术大学取得博士学位,后在多伦多大学Dalla Lana公共卫生学院从事博士后研究,先后访问过新加坡国立大学和约克大学。主要从事高维数据分析和个性化医疗等领域的研究。在SCIENCE CHINA-Mathematics和Statistics in Medicine等国际知名学术期刊上发表多篇论文。
报告人:张佳 西南财经大学 博士后
报告时间:12月5号上午 9:30-10:00
报告地点:腾讯会议 ID 504 790 537
报告题目:High Dimensional Elliptical Sliced Inverse Regression in non-Gaussian Distributions
摘要:Sliced inverse regression (SIR) is the most widely-used sufficient dimension reduction method due to its simplicity, generality and computational efficiency. However, when the distribution of the covariates deviates from the multivariate normal distribution, the estimation efficiency of SIR gets rather low, and the SIR estimator may be inconsistent and misleading, especially in high-dimensional setting. In this paper, we propose a robust alternative to SIR - called elliptical sliced inverse regression (ESIR) for analyzing high-dimensional, elliptically distributed data. There are wide applications of the elliptically distributed data, especially in finance and economics where the distribution of the data is often heavy-tailed. To tackle the heavy-tailed elliptically distributed covariates, we novelly utilize the multivariate Kendall's tau matrix in a framework of generalized eigenvalue problem in sufficient dimension reduction. Methodologically, we present a practical algorithm for our method. Theoretically, we investigate the asymptotic behavior of the ESIR estimator under high-dimensional setting. Simulation results show that ESIR significantly improves the estimation efficiency in heavy-tailed scenarios. Analysis of the Istanbul stock exchange data set also demonstrates the effectiveness of our proposed method. Moreover, ESIR can be easily extended to other sufficient dimension reduction methods and applied to non-elliptical heavy-tailed distributions.
个人简介:张佳,经济学博士。2019年6月在西南财经大学取得博士学位,同年进入西南财经大学从事博士后研究,博士后导师为常晋源教授。主要从事高维经验似然和充分降维等领域的研究。在CSDA、JMVA和JSPI等国际知名学术期刊上发表多篇论文。
报告人:王杨 上海交通大学 博士后
报告时间:12月5号上午 10:00-10:30
报告地点:腾讯会议 ID 504 790 537
报告题目:A Kernel Regression Model for Panel Count Data with Nonparametric Covariate Functions
摘要:Local kernel pseudo-partial likelihood is used for estimation in panel count model with nonparametric covariate functions. Estimator of the derivative of nonparametric covariate function is derived first and nonparametric function estimator is then obtained by integrating the derivative function. Under some regularity conditions, uniform consistency rates and pointwise asymptotic normality are obtained for the local derivative estimator. Moreover, the baseline function estimator is shown to be uniformly consistent. The demonstration of the asymptotic results relies strongly on the modern empirical theory, which not require the Poisson assumption. Simulation studies also show that the local derivative estimator performs well in finite-sample regardless of whether or not the Poisson assumption holds. We also apply the proposed methodology to analyze a clinical study on childhood wheezing.
个人简介:王杨,2014年本科毕业于信阳师范学院数学系;2016年硕士毕业于浙江大学数学系;2016年至今,在上海交通大学统计系攻读博士学位;2019年至2020年,在美国内布拉斯加州医学中心访学交流,师从面板计数数据领域的专家张殷教授。博士期间的研究方向包括面板计数数据、非参数统计分析、生存分析、临床试验研究。其中博士课题为医学面板计数数据的非参数统计分析。随着电子病历数据的广泛应用,面板计数数据在临床研究应用中很常见。面板计数数据作为一种只能在固定的随访时间点上观测的复发性事件数据,通常被用来研究试验因素的固定效应,但是在临床试验中,试验因素的效应往往是随时间变化或者是非线性。此时,固定效应模型就会导致效应估计的偏差,而非参数效应模型能够较好的分析这种时变效应或者非线性效应。因此,针对医学面板计数数据建立非参数效应模型尤为关键,能够为临床工作者提供更为准确的医学指导。博士期间以第一作者完成两篇文章,一篇于2019年被Statistica Sinica接受,另一篇在Biometrics二审中。与医生等合作完成6篇文章,其中4篇已经发表,2篇在审稿中。
报告人:张树雄 北京师范大学 博士
报告时间:12月5号上午 10:30-11:00
报告地点:腾讯会议 ID 504 790 537
报告题目:On large deviation probabilities for empirical distribution of the branching random walk with heavy tails
摘要:
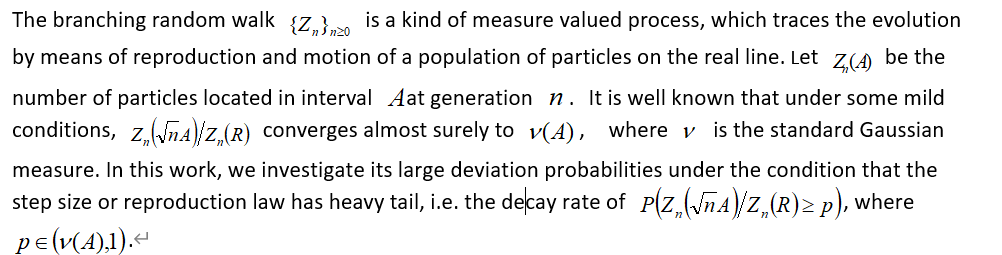
个人简介:张树雄, 概率论专业博士生。2016.09-2021.07年在北京师范大学学习(硕博连读),师从何辉教授。研究方向为分枝随机游动的大偏差理论,布朗运动,超过程等。


